Syngony

triclinic rhodonite

monoclinic vivianite

rhombic fayalite

tetragonal anatase

trigonal hematite

hexagonal beryl

cubic spessartine

Syngonia (from the Greek. Σύν "according to, together, near" + γωνία "angle";letters “Similarity”) - classification of crystallographic symmetry groups , crystals and crystal lattices depending on the coordinate system ( coordinate frame ); symmetry groups with a single coordinate system are combined into one syngony. Crystals belonging to the same system have similar angles and edges of unit cells .

The crystal system is a classification of crystals and crystallographic groups based on a set of symmetry elements that describe a crystal and belong to a crystallographic group.

Lattice system - classification of crystal lattices depending on their symmetry .

In the literature there is a confusion of all three concepts: syngonies ^[1] , the crystal system ^[2], and the lattice system ^[3] - which are often used as synonyms .

In the Russian-language literature, the term "grid system" is not used yet. Usually the authors mix this concept with the crystal system. In the book "Fundamentals of Crystallography" ^{[4], the} authors use the term "Grid syngony" (" By the symmetry of nodes, spatial gratings can be divided into seven categories, called syngony gratings "). In the same authors, syngonies are called systems (“ The most established classification of groups is their division into six systems based on the symmetry of the facet complexes ”).

Content

Syngony

Historically, the first classification of crystals was the division into syngonies, depending on the crystallographic coordinate system. The axes of symmetry of the crystal were chosen for the coordinate axes, and in their absence, the crystal edges. In the light of modern knowledge about the structure of crystals, the transmissions of the crystal lattice correspond to such directions, and the Brava cell translations in a standard installation are selected for the coordinate system. Depending on the ratio between the lengths of these broadcasts and the angles between them $\alpha ,\beta ,\gamma$ ${\ displaystyle \ alpha, \ beta, \ gamma}$ There are six different syngonies, which fall into three categories depending on the number of equal lengths of broadcasts:

Lower category (all broadcasts are not equal to each other)
- Triclinic : $a\neq b\neq c$ ${\ displaystyle a \ neq b \ neq c}$ , $\alpha \neq \beta \neq \gamma \neq 90^{\circ }$ ${\ displaystyle \ alpha \ neq \ beta \ neq \ gamma \ neq 90 ^ {\ circ}}$
- Monoclinic : $a\neq b\neq c$ ${\ displaystyle a \ neq b \ neq c}$ , $\alpha =\gamma =90^{\circ },\beta \neq 90^{\circ }$ ${\ displaystyle \ alpha = \ gamma = 90 ^ {\ circ}, \ beta \ neq 90 ^ {\ circ}}$
- Rhombic : $a\neq b\neq c$ ${\ displaystyle a \ neq b \ neq c}$ , $\alpha =\beta =\gamma =90^{\circ }$ ${\ displaystyle \ alpha = \ beta = \ gamma = 90 ^ {\ \ circ}}$

Middle category (two of the three broadcasts are equal)
- Tetragonal : $a=b\neq c$ ${\ displaystyle a = b \ neq c}$ , $\alpha =\beta =\gamma =90^{\circ }$ ${\ displaystyle \ alpha = \ beta = \ gamma = 90 ^ {\ \ circ}}$
- Hexagonal : $a=b\neq c$ ${\ displaystyle a = b \ neq c}$ , $\alpha =\beta =90^{\circ },\gamma =120^{\circ }$ ${\ displaystyle \ alpha = \ beta = 90 ^ {\ circ}, \ gamma = 120 ^ {\ circ}}$

Highest category (all broadcasts are equal)
- Cubic : $a=b=c$ ${\ displaystyle a = b = c}$ , $\alpha =\beta =\gamma =90^{\circ }$ ${\ displaystyle \ alpha = \ beta = \ gamma = 90 ^ {\ \ circ}}$

Crystal System

The division into crystalline systems is performed depending on the set of symmetry elements describing the crystal . Such a division leads to seven crystalline systems, two of which — trigonal (with one axis of the 3rd order) and hexagonal (with one axis of the 6th order) —are an elementary cell of the same shape and therefore belong to the same hexagonal syngony. It is sometimes said that hexagonal syngony is divided into two subingony ^[5] or hyposingonia. ^[6]

Crystal systems are also divided into three categories, depending on the number of higher order axes (higher than second order axes).

Possible in three-dimensional space crystalline systems with symmetry elements defining them, that is, symmetry elements, the presence of which is necessary for classifying a crystal or a point group to a specific crystalline system:

Lower category (no higher order axes)
- Triclinic : no symmetry or only center of inversion ${\overline {1}}$ ${\ displaystyle {\ overline {1}}}$
- Monoclinic : one axis $2$ ${\ displaystyle 2}$ th order and / or plane of symmetry $m$ ${\ displaystyle m}$
- Rhombic : three mutually perpendicular axes $2$ ${\ displaystyle 2}$ th order and / or plane of symmetry $m$ ${\ displaystyle m}$ (the direction of the plane of symmetry is considered perpendicular to it)
Middle category (one axis of higher order)
- Tetragonal : one axis $four$ ${\ displaystyle 4}$ th order or ${\overline {4}}$ ${\ displaystyle {\ overline {4}}}$
- Trigonal : one axis $3$ ${\ displaystyle 3}$ th order
- Hexagonal : one axis $6$ ${\ displaystyle 6}$ th order or ${\overline {6}}$ ${\ displaystyle {\ overline {6}}}$
Highest category (several higher order axes)
- Cubic : four axes $3$ ${\ displaystyle 3}$ th order

The crystal system of a space group is determined by the system of the corresponding point group. For example, the groups Pbca, Cmcm, Immm, Fddd ( class mmm) belong to the rhombic system.

The modern definition of the crystal system (applicable not only to ordinary three-dimensional groups, but also for spaces of any dimensions) assigns point groups (and space groups derived from them) to the same crystal system, if these groups can be combined with the same Bravais lattices. For example, the mm2 and 222 groups both belong to the rhombic system, since for each of them there are space groups with all types of rhombic lattices (Pmm2, Cmm2, Imm2, Fmm2 and P222, C222, I222, F222), at the same time groups 32 and 6 does not belong to the same crystal system, since for group 32, primitive and double-centered hexagonal cells (groups P321 and R32) are permissible, while group 6 is combined only with a primitive hexagonal cell (there is a group P 6 , but R 6 does not exist).

Lattice system

Describes the types of crystal lattices. In short: gratings are of the same type, if their point symmetry groups (when treating gratings as geometric objects) are the same. Such point groups, which describe the symmetry of the lattice, are called holohedry . ^[7]

In total, there are seven lattice systems, which, similar to the previous classifications (syngony and crystal system), are divided into three categories.

Lower category (all broadcasts are not equal to each other)
- Triclinic : $a\neq b\neq c$ ${\ displaystyle a \ neq b \ neq c}$ , $\alpha \neq \beta \neq \gamma \neq 90^{\circ }$ ${\ displaystyle \ alpha \ neq \ beta \ neq \ gamma \ neq 90 ^ {\ circ}}$
- Monoclinic : $a\neq b\neq c$ ${\ displaystyle a \ neq b \ neq c}$ , $\alpha =\gamma =90^{\circ },\beta \neq 90^{\circ }$ ${\ displaystyle \ alpha = \ gamma = 90 ^ {\ circ}, \ beta \ neq 90 ^ {\ circ}}$
- Rhombic : $a\neq b\neq c$ ${\ displaystyle a \ neq b \ neq c}$ , $\alpha =\beta =\gamma =90^{\circ }$ ${\ displaystyle \ alpha = \ beta = \ gamma = 90 ^ {\ \ circ}}$

Middle category
- Tetragonal : $a=b\neq c$ ${\ displaystyle a = b \ neq c}$ , $\alpha =\beta =\gamma =90^{\circ }$ ${\ displaystyle \ alpha = \ beta = \ gamma = 90 ^ {\ \ circ}}$
- Hexagonal : $a=b\neq c$ ${\ displaystyle a = b \ neq c}$ , $\alpha =\beta =90^{\circ },\gamma =120^{\circ }$ ${\ displaystyle \ alpha = \ beta = 90 ^ {\ circ}, \ gamma = 120 ^ {\ circ}}$
- Rhombohedral : $a=b=c$ ${\ displaystyle a = b = c}$ , $\alpha =\beta =\gamma <120^{\circ }\neq 90^{\circ }$ ${\ displaystyle \ alpha = \ beta = \ gamma <120 ^ {\ \}} \ neq 90 ^ {\ circ}}$

Highest category (all broadcasts are equal)
- Cubic : $a=b=c$ ${\ displaystyle a = b = c}$ , $\alpha =\beta =\gamma =90^{\circ }$ ${\ displaystyle \ alpha = \ beta = \ gamma = 90 ^ {\ \ circ}}$

Do not confuse the rhombohedral lattice system with the trigonal crystal system. Crystals of the rhombohedral lattice system always belong to the trigonal crystal system, but trigonal crystals can belong to both rhombohedral and hexagonal lattice systems. For example, the groups R 3 and P321 (both of the trigonal crystal system) belong to different lattice systems (rhombohedral and hexagonal, respectively).

General definition applicable to spaces of any dimensions - Lattices are of the same type if they are combined with the same point groups. For example, all rhombic lattices (rhombic P, rhombic C, rhombic I, and rhombic F) are of the same type, since they are combined with the point groups 222, mm2 and mmm, forming space groups P222, Pmm2, Pmmm; C222, Cmm2, Cmmm; I222, Imm2, Immm; F222, Fmm2, Fmmm. At the same time, the cells of the hexagonal system (primitive P and double-centered R) correspond to different lattice systems: both are combined with point groups of the trigonal crystal system, but only the primitive cell is combined with the groups of the hexagonal system (there are groups P6, P 6 , P6 / m, P622, P6mm, P 6 m2, P6 / mmm, but there are no groups R6, R 6 , R6 / m, R622, R6mm, R 6 m2, R6 / mmm).

The relationship between the syngony, the crystal system, and the lattice system in three-dimensional space is given in the following table:

Syngony	Crystal system	Point groups	The number of space groups	Grate Bravais ^[8]	Grid system	Golohedria
Triclinic		1, 1	2	aP	Triclinic	one
Monoclinic		2, m, 2 / m	13	mP, mS	Monoclinic	2 / m
Rhombic		222, mm2, mmm	59	oP, oS, oI, oF	Rhombic	mmm
Tetragonal		4, 4 , 422, 4mm, 4 2m, 4 / m, 4 / mmm	68	tP, tI	Tetragonal	4 / mmm
Hexagonal	Trigonal	3, 3 , 32, 3m, 3 m	7	hR	Rhombohedral	3 m
	Trigonal	3, 3 , 32, 3m, 3 m	18	hP	Hexagonal	6 / mmm
	Hexagonal	6, 6 , 622, 6mm, 6 m2, 6 / m, 6 / mmm	27	hP	Hexagonal	6 / mmm
Cubic		23, m 3 , 4 3m, 432, m 3 m	36	cP, cI, cF	Cubic	m 3 m
Total: 6	7	32	230	14	7

Point groups overview

Crystal system	point group / symmetry class	Schoenflies symbol	International symbol	Shubnikov symbol	Type of
triclinic	monohedral	C ₁	$1\$ ${\ displaystyle 1 \}$	$1\$ ${\ displaystyle 1 \}$	enantiomorphic polar
triclinic	pinacoid	C _i	${\bar {1}}$ ${\ displaystyle {\ bar {1}}}$	${\tilde {2}}$ ${\ displaystyle {\ tilde {2}}}$	centrosymmetric
monoclinic	dihedral axial	C ₂	$2\$ ${\ displaystyle 2 \}$	$2\$ ${\ displaystyle 2 \}$	enantiomorphic polar
	non-axial dihedral (domatic)	C _s	$m\$ ${\ displaystyle m \}$	$m\$ ${\ displaystyle m \}$	polar
	prismatic	C _2h	$2/m\$ ${\ displaystyle 2 / m \}$	$2:m\$ ${\ displaystyle 2: m \}$	centrosymmetric
Rhombic	rhombo-tetrahedral	D ₂	$222\$ ${\ displaystyle 222 \}$	$2:2\$ ${\ displaystyle 2: 2 \}$	enantiomorphic
	rhombo- pyramidal	C _2v	$mm2\$ ${\ displaystyle mm2 \}$	$2\cdot m\$ ${\ displaystyle 2 \ cdot m \}$	polar
	rhombic bipyramidal	D _2h	$mmm\$ ${\ displaystyle mmm \}$	$m\cdot 2:m\$ ${\ displaystyle m \ cdot 2: m \}$	centrosymmetric
Tetragonal	tetragonal pyramidal	C ₄	$4\$ ${\ displaystyle 4 \}$	$4\$ ${\ displaystyle 4 \}$	enantiomorphic polar
	tetragonal tetrahedral	S ₄	${\bar {4}}$ ${\ displaystyle {\ bar {4}}}$	${\tilde {4}}$ ${\ displaystyle {\ tilde {4}}}$
	tetragonal-dipyramidal	C _4h	$4/m\$ ${\ displaystyle 4 / m \}$	$4:m\$ ${\ displaystyle 4: m \}$	centrosymmetric
	tetragonal trapezoidal	D ₄	$422\$ ${\ displaystyle 422 \}$	$4:2\$ ${\ displaystyle 4: 2 \}$	enantiomorphic
	dietragonal-pyramidal	C _4v	$4mm\$ ${\ displaystyle 4mm \}$	$4\cdot m\$ ${\ displaystyle 4 \ cdot m \}$	polar
	tetragonal-scalohedral	D _2d	${\bar {4}}2m\$ ${\ displaystyle {\ bar {4}} 2m \}$ or ${\bar {4}}m2$ ${\ displaystyle {\ bar {4}} m2}$	${\tilde {4}}\cdot m$ ${\ displaystyle {\ tilde {4}} \ cdot m}$
	dititragonal-dipyramidal	D _4h	$4/mmm\$ ${\ displaystyle 4 / mmm \}$	$m\cdot 4:m\$ ${\ displaystyle m \ cdot 4: m \}$	centrosymmetric
Trigonal	trigonal pyramidal	C ₃	$3$ ${\ displaystyle 3}$	$3\$ ${\ displaystyle 3 \}$	enantiomorphic polar
	rhombohedral	S ₆ (C _3i )	${\bar {3}}$ ${\ displaystyle {\ bar {3}}}$	${\tilde {6}}$ ${\ displaystyle {\ tilde {6}}}$	centrosymmetric
	trigonal trapezoidal	D ₃	$32\$ ${\ displaystyle 32 \}$ or $321\$ ${\ displaystyle 321 \}$ or $312\$ ${\ displaystyle 312 \}$	$3:2\$ ${\ displaystyle 3: 2 \}$	enantiomorphic
	ditrigonal pyramidal	C _3v	$3m\$ ${\ displaystyle 3m \}$ or $3m1\$ ${\ displaystyle 3m1 \}$ or $31m\$ ${\ displaystyle 31m \}$	$3\cdot m\$ ${\ displaystyle 3 \ cdot m \}$	polar
	di-trigonal scaleno	D _3d	${\bar {3}}m\$ ${\ displaystyle {\ bar {3}} m \}$ or ${\bar {3}}m1$ ${\ displaystyle {\ bar {3}} m1}$ or ${\bar {3}}1m$ ${\ displaystyle {\ bar {3}} 1m}$	${\tilde {6}}\cdot m$ ${\ displaystyle {\ tilde {6}} \ cdot m}$	centrosymmetric
Hexagonal	hexagonal-pyramidal	C ₆	$6\$ ${\ displaystyle 6 \}$	$6\$ ${\ displaystyle 6 \}$	enantiomorphic polar
	trigonal dipyramidal	C _3h	${\bar {6}}$ ${\ displaystyle {\ bar {6}}}$	$3:m\$ ${\ displaystyle 3: m \}$
	hexagonal dipyramidal	C _6h	$6/m\$ ${\ displaystyle 6 / m \}$	$6:m\$ ${\ displaystyle 6: m \}$	centrosymmetric
	hexagonal trapezoidal	D ₆	$622\$ ${\ displaystyle 622 \}$	$6:2\$ ${\ displaystyle 6: 2 \}$	enantiomorphic
	dihexagonal-pyramidal	C _6v	$6mm\$ ${\ displaystyle 6mm \}$	$6\cdot m\$ ${\ displaystyle 6 \ cdot m \}$	polar
	ditrigonal dipyramidal	D _3h	${\bar {6}}m2$ ${\ displaystyle {\ bar {6}} m2}$ or ${\bar {6}}2m$ ${\ displaystyle {\ bar {6}} 2m}$	$m\cdot 3:m\$ ${\ displaystyle m \ cdot 3: m \}$
	dihexagonal-dipyramidal	D _6h	$6/mmm\$ ${\ displaystyle 6 / mmm \}$	$m\cdot 6:m\$ ${\ displaystyle m \ cdot 6: m \}$	centrosymmetric
Cubic	tritetrahedral	T	$23\$ {\ displaystyle 23 \}	$3/2\$ ${\ displaystyle 3/2 \}$	enantiomorphic
	didodecahedral	T _h	$m{\bar {3}}\$ ${\ displaystyle m {\ bar {3}} \}$	${\tilde {6}}/2$ ${\ displaystyle {\ tilde {6}} / 2}$	centrosymmetric
	hexa tetrahedral	T _d	${\bar {4}}3m$ ${\ displaystyle {\ bar {4}} 3m}$	$3/{\tilde {4}}$ ${\ displaystyle 3 / {\ tilde {4}}}$
	trioctahedral	O	$432\$ ${\ displaystyle 432 \}$	$3/4\$ ${\ displaystyle 3/4 \}$	enantiomorphic
	hexactahedral	O _h	$m{\bar {3}}m$ ${\ displaystyle m {\ bar {3}} m}$	${\tilde {6}}/4$ ${\ displaystyle {\ tilde {6}} / 4}$	centrosymmetric

Lattice classification

Syngony	Type of centering cell Brava
Syngony	primitive	base centered	volume centered	facet centered	twice volume centered
Triclinic ( parallelepiped )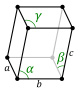
Monoclinic ( prism with parallelogram at the base)
Rhombic ( rectangular parallelepiped )
Tetragonal ( rectangular box with a square at the base)
Hexagonal ( prism with the base of a regular centered hexagon)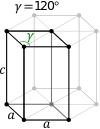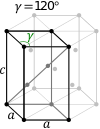
Cubic ( cube )

History

The first geometric classification of crystals was given independently by Christian Weiss and Friedrich Moos at the beginning of the 19th century. Both scientists classified crystals according to the symmetry of their external shape (faceting). In this case, Weiss actually introduces the concept of a crystallographic axis (axis of symmetry). According to Weiss, “The axis is the line that dominates the entire figure of the crystal, since around it all the parts are arranged in a similar way and relative to it they correspond to each other mutually” ^[12] . In his work “A visual representation of the natural divisions of crystallization systems,” Weiss classified crystals according to the presence of axes into four large sections of crystalline forms, “crystallization systems” corresponding to the modern concept of syngony ^[13] . In brackets are the modern names.

Section 1 - “correct”, “spherohedral”, “equiaxial”, “equal” (cubic) system: three dimensions are the same, forming right angles between themselves.
- subsection homospheric system (crystals of symmetry m 3 m)
- subsection of hemisphere-hedron system (crystals of symmetry 432, 43m and m 3 )
Section 2 - “four-membered” (tetragonal) system: the axes form between themselves right angles, the two axes are equal to each other and not the third.
Section 3 - “two-member” system: all three axes are unequal and form right angles between themselves.
- subdivision "two-and-two-member" (rhombic) system
- subdivision “two-and-term” (monoclinic) system
- subsection "one-and-one-term" (triclinic) system
Section 4 - one unequal axis is perpendicular to three equal axes forming angles of 120 ° between each other.
- subsection "six-membered" (hexagonal) system:
- subdivision "three-and-trichinas" or "rhombohedral" (trigonal) system:

For the monoclinic and triclinic syngony, Weiss used a rectangular coordinate system (modern crystallographic coordinate systems for these syngonies are oblique).

Around the same time, Friedrich Moos developed the concept of crystalline systems ^[14] . Each system is characterized by the simplest, “basic form” of faces, from which all other forms of this system can be derived. Thus, Moos obtained the following four systems:

1. Rhombohedral system (hexagonal syngony). The main form is a rhombohedron.
2. Pyramidal system (tetragonal syngony). The main form is the tetragonal bipyramid.
3. The dessular system (cubic syngony). The main forms are a cube and an octahedron.
4. Prismatic system (rhombic syngony). The main form is the rhombic bipyramid.
- Hemiprimatic subsystem (monoclinic syngony)
- Tetaropismatic subsystem (triclinic syngony)

In both classifications, Weiss and Moos distinguishes only four systems, although all six syngonies are listed, they consider only the monoclinic and triclinic syngonies as rhombic subsystems. According to his own statement, Mohs developed this concept in the years 1812-14, which was the subject of a dispute with Weiss about the priority of the discovery of crystalline systems. Unlike Weiss, Mohs pointed out the need for an oblique axis system for monoclinic and triclinic crystals.

His pupil Karl Friedrich Naumann finally developed and introduced kosoo-angled systems into crystallography. Nauman based the classification of crystallographic axes and angles between them, thus highlighting for the first time all six syngonies ^[15] ^[16] . Interestingly, as early as 1830, Nauman uses the names of syngonies that are identical or close to modern (the names tetragonal , hexagonal and rhombic were originally proposed by Breitgaupt).

1. Tesseral (from tessera - cube) - all three angles between the coordinate axes are straight, all three axes are equal.
2. Tetragonal - all three corners are right, two axes are equal.
3. Hexagonal - the only four-axis system: one unequal axis is perpendicular to three equal axes forming angles of 60 ° between themselves.
4. Rhombic - all three corners are straight, all axes are unequal.
5. Monoclinohedral - two right angles and one oblique.
6. Diclinhedral - two oblique angles and one straight.
7. Triclinohedral — all three angles are oblique.

Since at that time the theory of symmetry was only developing, an unusual diclinohedral (diclin) system appeared in the list of systems. Such a crystal system is in principle impossible in three-dimensional space, since the presence of the axis of symmetry always guarantees the presence of translations perpendicular to the axis, chosen for the coordinate axes. Diklinnaya system existed in crystallography for about half a century (although already in 1856 Dufrenois showed that this is only a special case of the triclinic system). In 1880, Dana in her famous book “The System of Mineralogy” ^[17] mentions “the so-called diklinnuyu system”, but at the same time notes that not a single natural or artificial crystal belonging to this system is known, and moreover, it has been mathematically proven that there are only six crystalline systems. Until the end of his life, Nauman himself believed in a diklinny syngony, and in the ninth edition of The Foundations of Mineralogy ^[18] , posthumously published in 1874, this syngony is still on the list, although Nauman observes that this system is found only in a few artificial salts, and further does not consider it.

The names of crystallographic syngonies from the authors of the XIX century

Author	Cubic	Tetragonal	Hexagonal	Rhombic	Monoclinic	Triclinic
Weiss	Regular, Spherical, Ball, Spheronomic, Equal-Axis, Equal	Quadruple, Dual and Uniaxial	Six-membered, three-and-uniaxial	Two-and-two-threaded, Single-and-uniaxial	Double-and-term	One and one term
Moos	Teselyarnaya, Tesselyarnaya	Pyramidal	Rhombohedral	Prismatic Orthotype	Hemiprimatic, hemiortypical	Tetaroprimatic, anorotype
Breitgaupt		Tetragonal	Hexagonal	Rhombic	Hemirmbic	Tetartorhombic
Nauman	Tesseral	Tetragonal	Hexagonal	Rhombic, Anisometric	Monoclinhedral, Klinorombic	Triclinohedral, triclinometric
Gausman	Isometric	Monodimetric	Monotrimetric	Trimetric, Orthorhombic	Klinorombicheskaya, Orthorhombic	Klinoromboidicheskaya
Miller 1839	Octahedral	Pyramidal	Rhombohedral	Prismatic	Oblique prismatic	Double-oblique-prismatic
Gadolin	Correct	Square	Hexagonal	Rhombic	Monoclinohedral	Triclinohedral
Other authors	Tetrahedral (Bedan), Cubic (Dufrenois)	Dimetric		Twofold (Quenstedt)	Monoclinometric (Frankenheim), Augitovaya (Geidinger)	Triclinic (Frankenheim), Anortic (Geidinger)

For the first time, the division into seven crystallographic systems was given in 1850 in the work of Auguste Brava “Memoir on systems of points correctly distributed on a plane or in space” ^[19] . In fact, this is the first division based on symmetry elements, and not on coordinate systems. Therefore, all previous classifications correspond to today's definition of a syngony, while the Bravais classification is a classification according to crystalline systems (strictly speaking, lattice systems).

Depending on their symmetry, Bravais divides lattices into 7 systems (classes of aggregates).

1. Three-Quad (Cubic System)
2. Six (hexagonal system)
3. Quadruple (tetragonal system)
4. Triple (rhombohedral system)
5. Triple (rhombic system)
6. Dual (monoclinic system)
7. Asymmetric (triclinic system)

At the same time, Bravais himself notes that Haüy also divided the lattices of the hexagonal system (according to Naumann’s classification) “into crystals generated by a regular hexagonal prism, and crystals generated by a rombohedron nucleus”.

Classification of groups in multidimensional spaces

In the second half of the 20th century, crystallographic groups were studied and classified in four-dimensional, five-dimensional and six-dimensional spaces. With increasing dimension, the number of groups and classes increases significantly ^[20] . The number of enantiomorphic pairs is indicated in parentheses.

Space dimension:	one	2	3	four	five	6
Number of syngonies	one	four	6	23 (+6)	32	91
Number of lattice systems	one	four	7	33 (+7)	57	220
The number of crystalline systems	one	four	7	33 (+7)	59	251
The number of lattices Brava	one	five	14	64 (+10)	189	841
The number of point groups	2	ten	32	227 (+44)	955	7103
The number of space groups	2	17	219 (+11)	4783 (+111)	222018 (+79)	28927915 (+?) ^[21]

In four-dimensional space, the unit cell is defined by four sides ( $a, b, c, d$ ${\ displaystyle a, b, c, d}$ ) and six corners between them ( $\alpha ,\beta ,\gamma ,\delta ,\epsilon ,\zeta$ ${\ displaystyle \ alpha, \ beta, \ gamma, \ delta, \ epsilon, \ zeta}$ ). The following relationships between them define 23 syngonies:

Hexaclinic: $a\neq b\neq c\neq d,\alpha \neq \beta \neq \gamma \neq \delta \neq \epsilon \neq \zeta \neq 90^{\circ }$ ${\ displaystyle a \ neq b \ neq c \ neq d, \ alpha \ neq \ beta \ neq \ gamma \ neq \ delta \ neq \ epsilon \ neq \ zeta \ neq 90 ^ {\ circ}}$
Triclinic: $a\neq b\neq c\neq d,\alpha \neq \beta \neq \gamma \neq 90^{\circ },\delta =\epsilon =\zeta =90^{\circ }$ ${\ displaystyle a \ neq b \ neq c \ neq d, \ alpha \ neq \ beta \ neq \ gamma \ neq 90 ^ {\ circ}, \ delta = \ epsilon = \ zeta = 90 ^ {\ circ}}$
Diklinnaya: $a\neq b\neq c\neq d,\alpha \neq 90^{\circ },\beta =\gamma =\delta =\epsilon =90^{\circ },\zeta \neq 90^{\circ }$ ${\ displaystyle a \ neq b \ neq c \ neq d, \ alpha \ neq 90 ^ {\ circ}, \ beta = \ gamma = \ delta = \ epsilon = 90 ^ {\ circ}, \ zeta \ neq 90 ^ {\ circ}}$
Monoclinic: $a\neq b\neq c\neq d,\alpha \neq 90^{\circ },\beta =\gamma =\delta =\epsilon =\zeta =90^{\circ }$ ${\ displaystyle a \ neq b \ neq c \ neq d, \ alpha \ neq 90 ^ {\ circ}, \ beta = \ gamma = \ delta = \ epsilon = \ zeta = 90 ^ {\ circ}}$
Orthogonal: $a\neq b\neq c\neq d,\alpha =\beta =\gamma =\delta =\epsilon =\zeta =90^{\circ }$ ${\ displaystyle a \ neq b \ neq c \ neq d, \ alpha = \ beta = \ gamma = \ delta = \ epsilon = \ zeta = 90 ^ {\ circ}}$
Tetragonal monoclinic: $a\neq b=c\neq d,\alpha \neq 90^{\circ },\beta =\gamma =\delta =\epsilon =\zeta =90^{\circ }$ ${\ displaystyle a \ neq b = c \ neq d, \ alpha \ neq 90 ^ {\ circ}, \ beta = \ gamma = \ delta = \ epsilon = \ zeta = 90 ^ {\ circ}}$
Hexagonal monoclinic: $a\neq b=c\neq d,\alpha \neq 90^{\circ },\beta =\gamma =\delta =\epsilon =90^{\circ },\zeta =120^{\circ }$ ${\ displaystyle a \ neq b = c \ neq d, \ alpha \ neq 90 ^ {\ circ}, \ beta = \ gamma = \ delta = \ epsilon = 90 ^ {\ circ}, \ zeta = 120 ^ {\ circ}}$
Dietragonal diklinnaya: $a=d\neq b=c,\alpha =\zeta =90^{\circ },\beta =\epsilon \neq 90^{\circ },\gamma \neq 90^{\circ },\delta =180^{\circ }-\gamma$ ${\ displaystyle a = d \ neq b = c, \ alpha = \ zeta = 90 ^ {\ circ}, \ beta = \ epsilon \ neq 90 ^ {\ circ}, \ gamma \ neq 90 ^ {\ circ}, \ delta = 180 ^ {\ circ} - \ gamma}$
Ditrigonal Diklinnaya: $a=d\neq b=c,\alpha =\zeta =120^{\circ },\beta =\epsilon \neq 90^{\circ },\gamma \neq \delta \neq 90^{\circ },\cos \delta =\cos \beta -\cos \gamma$ ${\ displaystyle a = d \ neq b = c, \ alpha = \ zeta = 120 ^ {\ circ}, \ beta = \ epsilon \ neq 90 ^ {\ circ}, \ gamma \ neq \ delta \ neq 90 ^ { \ circ}, \ cos \ delta = \ cos \ beta - \ cos \ gamma}$
Tetragonal orthogonal: $a\neq b=c\neq d,\alpha =\beta =\gamma =\delta =\epsilon =\zeta =90^{\circ }$ ${\ displaystyle a \ neq b = c \ neq d, \ alpha = \ beta = \ gamma = \ delta = \ epsilon = \ zeta = 90 ^ {\ \ circ}}$
Hexagonal orthogonal: $a\neq b=c\neq d,\alpha =\beta =\gamma =\delta =\epsilon =90^{\circ },\zeta =120^{\circ }$ ${\ displaystyle a \ neq b = c \ neq d, \ alpha = \ beta = \ gamma = \ delta = \ epsilon = 90 ^ {\ circ}, \ zeta = 120 ^ {\ circ}}$
Dietragonal monoclinic: $a=d\neq b=c,\alpha =\gamma =\delta =\zeta =90^{\circ },\beta =\epsilon \neq 90^{\circ }$ ${\ displaystyle a = d \ neq b = c, \ alpha = \ gamma = \ delta = \ zeta = 90 ^ {\ circ}, \ beta = \ epsilon \ neq 90 ^ {\ circ}}$
Ditrigonal Monoclinic: $a=d\neq b=c,\alpha =\zeta =120^{\circ },\beta =\epsilon \neq 90^{\circ },\gamma =\delta \neq 90^{\circ },\cos \gamma =-\color {Black}{\tfrac {1}{2}}\cos \beta$ ${\ displaystyle a = d \ neq b = c, \ alpha = \ zeta = 120 ^ {\ circ}, \ beta = \ epsilon \ neq 90 ^ {\ circ}, \ gamma = \ delta \ neq 90 ^ {\ circ}, \ cos \ gamma = - \ color {Black} {\ tfrac {1} {2}} \ cos \ beta}$
Dietragonal orthogonal: $a=d\neq b=c,\alpha =\beta =\gamma =\delta =\epsilon =\zeta =90^{\circ }$ ${\ displaystyle a = d \ neq b = c, \ alpha = \ beta = \ gamma = \ delta = \ epsilon = \ zeta = 90 ^ {\ \ circ}}$
Hexagonal tetragonal: $a=d\neq b=c,\alpha =\beta =\gamma =\delta =\epsilon =90^{\circ },\zeta =120^{\circ }$ ${\ displaystyle a = d \ neq b = c, \ alpha = \ beta = \ gamma = \ delta = \ epsilon = 90 ^ {\ circ}, \ zeta = 120 ^ {\ circ}}$
Dihexagonal orthogonal: $a=d\neq b=c,\alpha =\zeta =120^{\circ },\beta =\gamma =\delta =\epsilon =90^{\circ }$ ${\ displaystyle a = d \ neq b = c, \ alpha = \ zeta = 120 ^ {\ circ}, \ beta = \ gamma = \ delta = \ epsilon = 90 ^ {\ circ}}$
Cubic orthogonal: $a=b=c\neq d,\alpha =\beta =\gamma =\delta =\epsilon =\zeta =90^{\circ }$ ${\ displaystyle a = b = c \ neq d, \ alpha = \ beta = \ gamma = \ delta = \ epsilon = \ zeta = 90 ^ {\ \ circ}}$
Octagonal: $a=b=c=d,\alpha =\gamma =\zeta \neq 90^{\circ },\beta =\epsilon =90^{\circ },\delta =180^{\circ }-\alpha$ ${\ displaystyle a = b = c = d, \ alpha = \ gamma = \ zeta \ neq 90 ^ {\ circ}, \ beta = \ epsilon = 90 ^ {\ circ}, \ delta = 180 ^ {\ circ} - \ alpha}$
Decagonal: $a=b=c=d,\alpha =\gamma =\zeta \neq \beta =\delta =\epsilon ,\cos \beta =-0.5-\cos \alpha$ ${\ displaystyle a = b = c = d, \ alpha = \ gamma = \ zeta \ neq \ beta = \ delta = \ epsilon, \ cos \ beta = -0.5- \ cos \ alpha}$
Dodecagonal: $a=b=c=d,\alpha =\zeta =90^{\circ },\beta =\epsilon =120^{\circ },\gamma =\delta \neq 90^{\circ }$ ${\ displaystyle a = b = c = d, \ alpha = \ zeta = 90 ^ {\ circ}, \ beta = \ epsilon = 120 ^ {\ circ}, \ gamma = \ delta \ neq 90 ^ {\ circ} }$
Di-isohexagonal orthogonal: $a=b=c=d,\alpha =\zeta =120^{\circ },\beta =\gamma =\delta =\epsilon =90^{\circ }$ ${\ displaystyle a = b = c = d, \ alpha = \ zeta = 120 ^ {\ circ}, \ beta = \ gamma = \ delta = \ epsilon = 90 ^ {\ circ}}$
Ikosagonalnaya: $a=b=c=d,\alpha =\beta =\gamma =\delta =\epsilon =\zeta ,\cos \alpha =-\color {Black}{\tfrac {1}{4}}$ ${\ displaystyle a = b = c = d, \ alpha = \ beta = \ gamma = \ delta = \ epsilon = \ zeta, \ cos \ alpha = - \ color {Black} {\ tfrac {1} {4}} }$
Hypercubic: $a=b=c=d,\alpha =\beta =\gamma =\delta =\epsilon =\zeta =90^{\circ }$ ${\ displaystyle a = b = c = d, \ alpha = \ beta = \ gamma = \ delta = \ epsilon = \ zeta = 90 ^ {\ circ}}$

The relationship between the syngony, the crystal system, and the lattice system in four-dimensional space is given in the following table ^[22] ^[23] . Asterisks indicate enantiomorphic systems. The number of enantiomorphic groups (or lattices) is indicated in parentheses.

room syngony	Syngony	Crystal system	room systems	The number of point groups	The number of space groups	The number of lattices Brava	Grid system
I	Hexaclinic		one	2	2	one	Hexaclinic P
II	Triclinic		2	3	13	2	Triclinic P, S
III	Diklinnaya		3	2	12	3	Diklinnaya P, S, D
IV	Monoclinic		four	four	207	6	Monoclinic P, S, S, I, D, F
V	Orthogonal	Axisless orthogonal	five	2	2	one	Orthogonal KU
		Axisless orthogonal	five	2	112	eight	Orthogonal P, S, I, Z, D, F, G, U
		Axial orthogonal	6	3	887	eight	Orthogonal P, S, I, Z, D, F, G, U
VI	Tetragonal monoclinic		7	7	88	2	Tetragonal monoclinic P, I
VII	Hexagonal Monoclinic	Trigonal Monoclinic	eight	five	9	one	Hexagonal Monoclinic R
		Trigonal Monoclinic	eight	five	15	one	Hexagonal Monoclinic P
		Hexagonal Monoclinic	9	7	25	one	Hexagonal Monoclinic P
Viii	Dietragonal diklinnaya *		ten	1 (+1)	1 (+1)	1 (+1)	Dietragonal diklinnaya P *
Ix	Ditrigonal Diklinnaya *		eleven	2 (+2)	2 (+2)	1 (+1)	Ditrigonal Diclinic P *
X	Tetragonal orthogonal	Inversion tetragonal orthogonal	12	five	7	one	Tetragonal orthogonal KG
		Inversion tetragonal orthogonal	12	five	351	five	Tetragonal orthogonal P, S, I, Z, G
		Rotary tetragonal orthogonal	13	ten	1312	five	Tetragonal orthogonal P, S, I, Z, G
Xi	Hexagonal orthogonal	Trigonal orthogonal	14	ten	81	2	Hexagonal orthogonal R, RS
		Trigonal orthogonal	14	ten	150	2	Hexagonal orthogonal P, S
		Hexagonal orthogonal	15	12	240	2	Hexagonal orthogonal P, S
Xii	Dietragonal monoclinic *		sixteen	1 (+1)	6 (+6)	3 (+3)	Dietragonal monoclinic P , S , D *
XIII	Ditrigonal monoclinic *		17	2 (+2)	5 (+5)	2 (+2)	Ditrigonal monoclinic P , RR
Xiv	Dietragonal orthogonal	Cryptodietragonal orthogonal	18	five	ten	one	Dietragonal orthogonal D
		Cryptodietragonal orthogonal	18	five	165 (+2)	2	Dietragonal orthogonal P, Z
		Dietragonal orthogonal	nineteen	6	127	2	Dietragonal orthogonal P, Z
Xv	Hexagonal tetragonal		20	22	108	one	Hexagonal Tetragonal P
XVI	Dihexagonal orthogonal	Crypto-ditrigonal orthogonal *	21	4 (+4)	5 (+5)	1 (+1)	Dihexagonal orthogonal G *
		Crypto-ditrigonal orthogonal *	21	4 (+4)	5 (+5)	one	Dihexagonal orthogonal P
		Dihexagonal orthogonal	23	eleven	20
		Ditrigonal Orthogonal	22	eleven	41
		Ditrigonal Orthogonal	22	eleven	sixteen	one	Dihexagonal orthogonal RR
XVII	Cubic orthogonal	Simple cubic orthogonal	24	five	9	one	Cubic orthogonal KU
		Simple cubic orthogonal	24	five	96	five	Cubic orthogonal P, I, Z, F, U
		Complex cubic orthogonal	25	eleven	366	five	Cubic orthogonal P, I, Z, F, U
XVIII	Octagon *		26	2 (+2)	3 (+3)	1 (+1)	Octagonal P *
XIX	Decagonal		27	four	five	one	Decagonal P
Xx	Dodecagonal *		28	2 (+2)	2 (+2)	1 (+1)	Dodecagonal P *
XXI	Di-isohexagonal orthogonal	Simple di-isohexagonal orthogonal	29	9 (+2)	19 (+5)	one	Di-isohexagonal orthogonal RR
		Simple di-isohexagonal orthogonal	29	9 (+2)	19 (+3)	one	Di-isohexagonal orthogonal P
		Difficult di-isohexagonal orthogonal	thirty	13 (+8)	15 (+9)	one	Di-isohexagonal orthogonal P
XXII	Ikosagonalnaya		31	7	20	2	Icosal P, SN
XXIII	Hypercubic	Octagonal hypercubic	32	21 (+8)	73 (+15)	one	Hypercubic P
		Octagonal hypercubic	32	21 (+8)	107 (+28)	one	Hypercubic Z
		Dodecagonal hypercubic	33	16 (+12)	25 (+20)	one	Hypercubic Z
Total:	23 (+6)	33 (+7)		227 (+44)	4783 (+111)	64 (+10)	33 (+7)

Notes

↑ Crystal family - Online Dictionary of Crystallography (Neopr.) . Archived March 21, 2013.
↑ Crystal system - Online Dictionary of Crystallography (Neopr.) . Archived March 21, 2013.
↑ Lattice system - Online Dictionary of Crystallography (Neopr.) . Archived April 29, 2013.
↑ Shubnikov A. V., Boky G. B., Flint E. E., Basics of Crystallography, Publishing House of the Academy of Sciences of the USSR, 1940
↑ "Yu. K. Egorov-Tismenko, G. P. Litvinskaya, Theory of Crystal Symmetry, GEOS, 2000. Chapter III. Coordinate Systems, Categories, Syngonies." (Neopr.) Archived April 29, 2013.
↑ Fedorov E.S., Course of crystallography. Ed. 3rd, 1901 online
↑ Holohedry - Online Dictionary of Crystallography (Neopr.) . Archived March 21, 2013.
↑ de Wolff et al., Nomenclature for crystal families, Bravais-lattice types and arithmetic classes, Acta Cryst. (1985). A41,278-280. online
↑ Weinstein B.K. Modern crystallography. Volume 1. Symmetry of crystals, methods of structural crystallography. Science, Moscow, 1979.
↑ Sirotin Yu.I., Shaskolskaya MP Basics of crystal physics. Science, Moscow, 1979.
↑ Flint E.E. A practical guide to geometric crystallography. 3rd ed, perarab. and add., Gosgeoltehizdat, Moscow, 1956.
↑ CS Weiss De indagando formarum crystallinarum charactere geometrico principali dissertatio. Lipsiae [Leipzig] 1809
↑ CS Weiss : Ueber die natürlichen Abtheilungen der Crystallisations Systeme. Abhandl. k. Akad. Wiss., Berlin 1814–1815, S. 290–336.
↑ Friedrich Mohs : Grund-Riß der Mineralogie. Erster Theil. Terminologie, Systematik, Nomenklatur, Charakteristik. Dresden 1822
↑ Carl Friedrich Naumann , Lehrbuch der Mineralogie Mineralogie, 1828 online
↑ Carl Friedrich Naumann , Lehrbuch der reinen und angewandten Krystallographie, 1830 online
↑ Edward Salisbury Dana, James Dwight Dana, A text-book of mineralogy, 1880 online
↑ Carl Friedrich Naumann, Elemente der mineralogie, 1874 online
↑ Bravais, A. (1850) Mémoire sur les systèmes formés par les points distribute régulièrement sur planes ou dans l'espace. Journal de L'Ecole Polytechnique.
↑ B. Souvignier: "Enantiomorphism of crystallographic groups". Acta Crystallographica Section A, vol.59, pp.210-220, 2003.
↑ The CARAT Homepage ( Undefeated ) . The circulation date was May 5, 2015. Part of the calculations in Souvignier (2003) for the six-dimensional space was based on the erroneous version of the CARAT program.
J EJW Whittaker, An atlas of hyperstereograms of the four-dimensional crystal classes. Clarendon Press (Oxford Oxfordshire and New York) 1985.
↑ H. Brown, R. Bülow, J. Neubüser, H. Wondratschek and H. Zassenhaus, Crystallographic Groups of Four-Dimensional Space. Wiley, NY, 1978.

Links

Glossary of terms on the website of the International Union of Crystallographers

[1] Crystal family - Online Dictionary of Crystallography (Neopr.) . Archived March 21, 2013.

[2] Crystal system - Online Dictionary of Crystallography (Neopr.) . Archived March 21, 2013.

[3] Lattice system - Online Dictionary of Crystallography (Neopr.) . Archived April 29, 2013.

[4] Shubnikov A. V., Boky G. B., Flint E. E., Basics of Crystallography, Publishing House of the Academy of Sciences of the USSR, 1940

[5] "Yu. K. Egorov-Tismenko, G. P. Litvinskaya, Theory of Crystal Symmetry, GEOS, 2000. Chapter III. Coordinate Systems, Categories, Syngonies." (Neopr.) Archived April 29, 2013.

[6] Fedorov E.S., Course of crystallography. Ed. 3rd, 1901 online

[7] Holohedry - Online Dictionary of Crystallography (Neopr.) . Archived March 21, 2013.

[8] Wolff et al., Nomenclature for crystal families, Bravais-lattice types and arithmetic classes, Acta Cryst. (1985). A41,278-280. online

[9] Weinstein B.K. Modern crystallography. Volume 1. Symmetry of crystals, methods of structural crystallography. Science, Moscow, 1979.

[10] Sirotin Yu.I., Shaskolskaya MP Basics of crystal physics. Science, Moscow, 1979.

[11] Flint E.E. A practical guide to geometric crystallography. 3rd ed, perarab. and add., Gosgeoltehizdat, Moscow, 1956.

[12] CS Weiss De indagando formarum crystallinarum charactere geometrico principali dissertatio. Lipsiae [Leipzig] 1809

[13] CS Weiss : Ueber die natürlichen Abtheilungen der Crystallisations Systeme. Abhandl. k. Akad. Wiss., Berlin 1814–1815, S. 290–336.

[14] Friedrich Mohs : Grund-Riß der Mineralogie. Erster Theil. Terminologie, Systematik, Nomenklatur, Charakteristik. Dresden 1822

[15] Carl Friedrich Naumann , Lehrbuch der Mineralogie Mineralogie, 1828 online

[16] Carl Friedrich Naumann , Lehrbuch der reinen und angewandten Krystallographie, 1830 online

[17] Edward Salisbury Dana, James Dwight Dana, A text-book of mineralogy, 1880 online

[18] Carl Friedrich Naumann, Elemente der mineralogie, 1874 online

[19] Bravais, A. (1850) Mémoire sur les systèmes formés par les points distribute régulièrement sur planes ou dans l'espace. Journal de L'Ecole Polytechnique.

[20] B. Souvignier: "Enantiomorphism of crystallographic groups". Acta Crystallographica Section A, vol.59, pp.210-220, 2003.

[21] The CARAT Homepage ( Undefeated ) . The circulation date was May 5, 2015. Part of the calculations in Souvignier (2003) for the six-dimensional space was based on the erroneous version of the CARAT program.

[22] J EJW Whittaker, An atlas of hyperstereograms of the four-dimensional crystal classes. Clarendon Press (Oxford Oxfordshire and New York) 1985.

[23] H. Brown, R. Bülow, J. Neubüser, H. Wondratschek and H. Zassenhaus, Crystallographic Groups of Four-Dimensional Space. Wiley, NY, 1978.

Syngony

Content

Syngony

Crystal System

Lattice system

Point groups overview

Lattice classification

History

Classification of groups in multidimensional spaces

See also

Notes

Links

More articles: